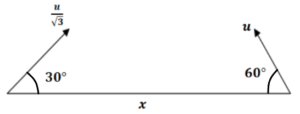◊ TWO DIMENSIONAL MOTION
In two-dimensional motion, the particle moves in a plane. Two-dimensional motion is a combination of two one-dimensional motions which is a mutually perpendicular direction.
i.e. two-dimensional motion can be resolved in two linear motions in two mutually perpendicular directions. Motion in one direction is independent of motion in another mutually perpendicular direction.
For example, we know that
similarly for
And so on…., means any formula in one dimension can use in two dimensions separately ( here in the x-axis and y-axis)
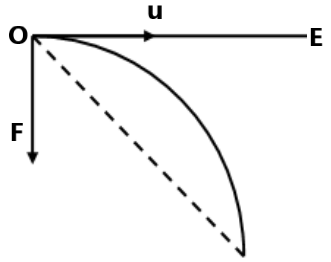
Exercise(1) A body starts from the origin with an acceleration of 6 m/s² along the x-axis and 8 m/s² along the y-axis. Find the distance from the origin after 4 sec. (80 m) (2) A body of mass 2 kg has an initial velocity of 3 m/s along OE and it is subjected to a force of 4N in OF direction perpendicular to OE. Find the displacement of the body from O after 4 sec. (20 cm)
(3) A particle moves in the x-y plane in such a way that its coordinates x and y vary with time as x=t³-32t and y=5t²+12. Here x and y are in meters and t in seconds. Find the position, velocity, and acceleration of the particle at t=3 sec. (4) A particle starts from the origin at t=0 with a velocity (a) what is the y coordinate of the particle at the instant its x coordinate is 84 m? ( 36 m) |
◊PROJECTILE MOTION
It is an example of two-dimensional motion under gravity ( acceleration is always -y-axis)
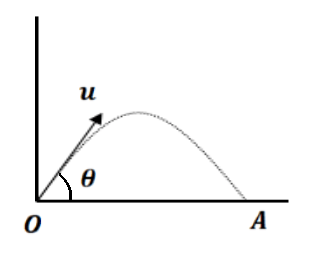
Angular Projection
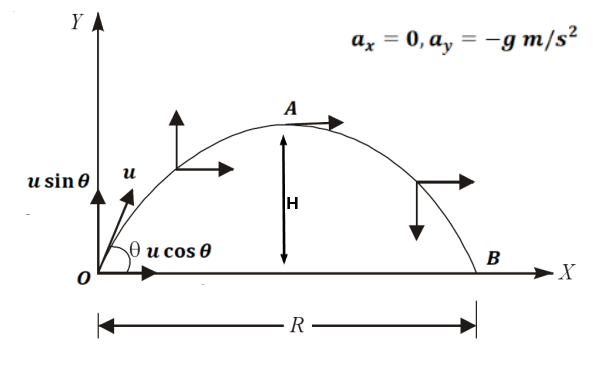
Let at t=0 , particle is at origin, initial velocity=u and angle of projection from horizontal=θ are given
Time remains in the air
We know that
take along Y-axis ( O to B)
Horizontal displacement traveled by the particle
take along x-axis (O to B)
Maximum vertical height from the launching point
We know that
take along y-axis ( from O to A)
We know that
Here R will be the maximum, when
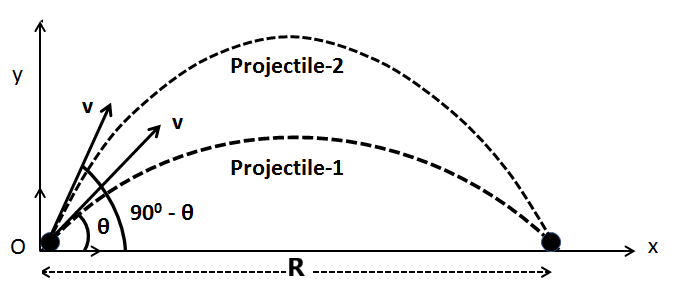
We know that range of projectile for angular projection is
Let for range will be
and for range will be
from (1) and (2)
i.e. for Range will be same
Note that in this case time of flight of both projectiles will be different
Let at any time t , particle is at P on trajectory whose co-ordinate is (x,y)
Now
Along x-axis
Along y-axis
From (1), we get
this is the equation of parabola i.e. the trajectory of the projectile is parabola
Now,
We know that
Now at time t,
Its magnitude is
Its direction is along tangent to the path at any instant
From fig
NOTE:- In projectile motion ( ground to ground) a body returns to the ground at the same angle and with the same speed at which it was projected

Numerical(1) A cricket ball is thrown at a speed of 28 m/s in a direction 30° above the horizontal. Calculate (a) Maximum height,(b) Time of flight, and (c) Horizontal range (10m, 2.9 sec, 63.3 m) (2) A body is projected with a velocity of 30° with the vertical. Find the maximum height, range, and Time of flight. (34.44 m, 5.3 m, 79.53 m) (3) A projectile has a range of 16 m and reaches a maximum height of 3 m. Calculate the angle at which the projectile is fired. (37°) (4) A football is kicked at an angle of 37° with a velocity of 20 m/s. calculate (a) The maximum height (b) The time of flight (c) How far away it hits the ground. (7.35 m, 2.45 sec, 39.2 m) (5) An athlete executing a long jump leaves the ground at an angle of 30° and travels 8.9 m. What was the take-off speed? ( 10 m/s) (6) Find the angle of projection for which the horizontal range and the maximum height are equal. (tanθ=4) (7) The velocity at the maximum height of a projectile is half its initial velocity of projection u. What is the horizontal range of the projectile? (8) Prove that (9) Find the average velocity from O to A (ucosθ)
|
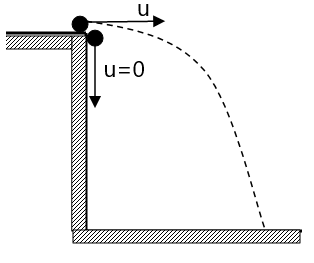
Horizontal Projection
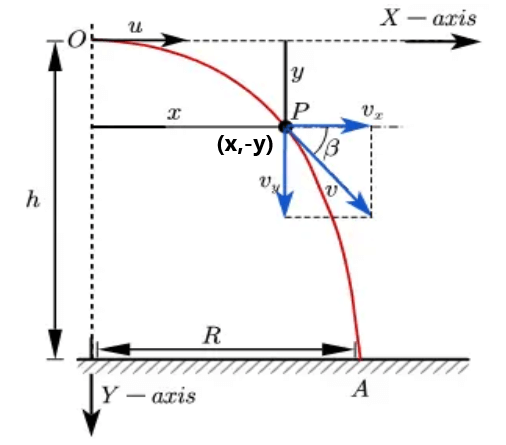
We know that
Along y-axis
We know that
Along x-axis
Let at any time t , particle is at P on trajectory whose coordinate is (x,-y)
Now Along x-axis
and along y-axis
This is the equation of a parabola
We know that
After time t
and
∴ Velocity at time t is given by
From fig
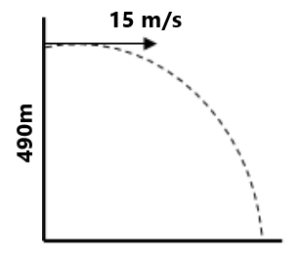
Numerical(1) Find the time taken by the stone to reach the ground, and the speed with which it hits the ground? ( 10 sec, 99.1 m/s)
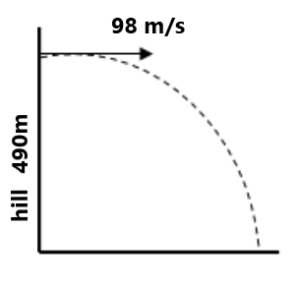
(2) Find (a) the time taken to reach the ground (b) The horizontal distance to the target from the hill and (c) the velocity ( magnitude and direction ) with which the projectile hits the ground) (10 sec, 980m, 138.6 m/s, 45°)
(3) A body is projected horizontally from the top of a cliff with a velocity 19.6 m/s. What time elapses before horizontal and vertical velocities become equal? (2 sec) (4) If both particles initiate at the same time. Show that the vertical distance covered by both will be the same in the same time interval.
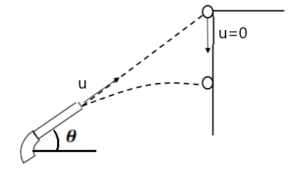
(5) Will the bullet hit the object? (Yes)
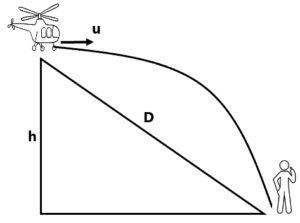
(6) A helicopter on a flood relief mission flying horizontally with a speed u at an altitude h, has to drop a flood packet for a victim standing on the ground. At what distance from the victim should the food packet be dropped?
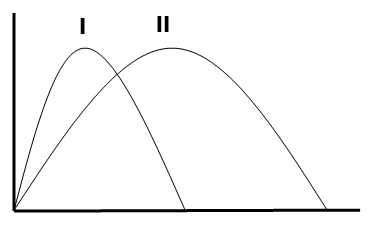
(7) A particle is projected with a velocity of 10 m/s at an angle of θ=37° with the horizontal. Find (a) Velocity of the particle after 1 sec. (4√5 m/s) (8) Which of the path has more time (same)
(9) A grasshopper can jump up to a height of h. Find the maximum distance through which it can jump along the horizontal ground. (2h) (10) Two projectiles A and B are projected horizontally from the top of a tower with speed |
Projectile motion on an inclined plane
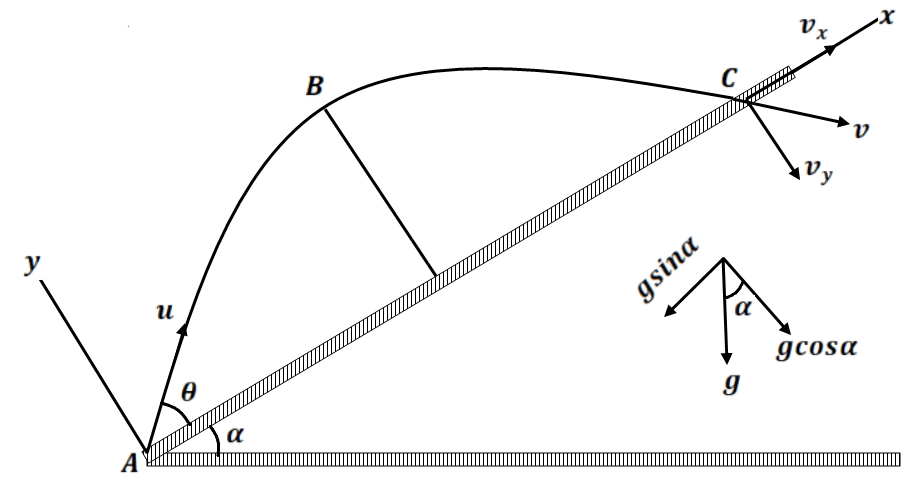
In this case, we should take the x-axis along the plane and the y-axis is along perpendicular to the plane.
i.e. acceleration due to gravity along the x-axis is and along the y-axis is
We know that
Take along y axis
We know that
Take along y axis from A to B
We know that
take along x-axis
For the maximum value of R, sin(2θ+α) must be the maximum
( This is the bisecting angle between the vertical and plane, i.e. we can say that the Range on the inclined plane is maximum when the direction of projection bisects the angle between the verticals and the inclined plane)
and
We know that
Then x and y components of the velocity of the projectile at any time t will be
NOTE:-
a. Time of ascent =Time of descent
b. The time of flight and maximum height are the same in both cases when particles are projected down in the inclined plane and projected up the inclined plane.
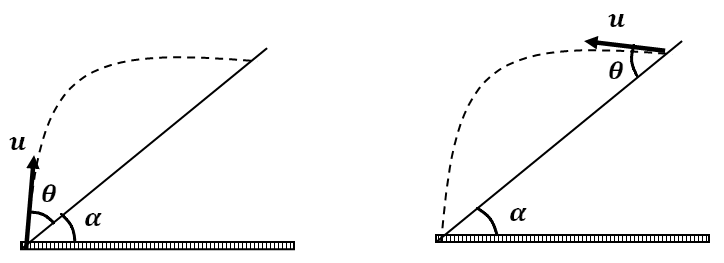
here in the second case
For maximum range angle should be and
miscellaneous exercise(1) A ball is projected horizontally with a speed v from the top of a plane inclined at an angle 45° with the horizontal. How far from the point of projection with the ball strike the plane? ( (2) (3) The velocity of projection of a projectile is given by (a) Time of flight (4) A stone is thrown with a velocity u at an angle θ with horizontal. Find its speed when it makes an angle β with the horizontal. (5) Find the two directions of projection to give a range of 45 m. If the projection speed is 30 m/s. (g=10m/s²) (15° or 75°) (6) A particle is projected with a speed of 40 m/s so that the projectile can hit the target at p( 80m,40m). Find the angle of projection. (7) A projectile is projected so as to just clear a wall of height 6m at a distance of 3m from the point of projection. The particle falls on the horizontal ground at a distance of 12 m from the wall. Find the angle of the projection. (8) The equation of the trajectory of a particle is given as in verticle plane. (a) Angle of projection (60°) (9) From the top of a 50 m high tower a stone is projected with a speed of 10 m/s, at an angle of 37° with horizontal .find (a) Velocity after 3 sec (10) A particle is projected with an initial speed of 20 m/s at an angle of projection 30° with the horizontal from a tower of height 40 m. Find the (g=10m/s²) (a) Time of flight (4 sec) (11) A projectile is projected with initial speed of 20 m/s at an angle of 30° with the horizontal as shown in from a tower of height 40 m (g=10m/s²) Find
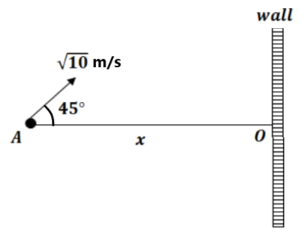
(a) Time of flight ( 2sec) (12) A jet of water is projected at an angle θ=45° with the horizontal from point A which is situated at a distance x=OA=(a) 1/2 m, (b) 2 m from a vertical wall. If the speed of projection is √10 m/s, find point P of striking the water jet with the vertical wall.
(13) The equation of projectile is (14) A large number of bullets are fired in all directions at the same speed u. What is the maximum area on the ground on which these bullets will spread? (15) A projectile is thrown with an initial velocity of (16) Two particles are separated at a horizontal distance x as shown in the figure.
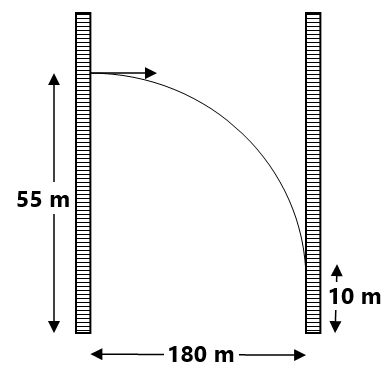
They are projected at the same time with different initial speeds. Find the time after which the horizontal displacement between the particles becomes zero. (x/4) (17) A body is projected horizontally from the top of a tower with an initial velocity of 18 m/s. It hits the ground at an angle of 45°. What is the vertical component of velocity when it strikes the ground? (18 m/s) (18) An object is thrown between two tall buildings 180 m from each other. The object thrown horizontally from a window 55 m above the ground from one building strikes a window 10 m above the ground in another window. Find out the speed of the projection. (60 m/s)
(19) A fighter plane moving with a speed of 50√2 m/s upward at an angle of 45° with the vertical released a bomb when it was at a height of 1000 m from ground. Find (a) The time of flight ( 20 sec) (20) Two stones A and B are projected simultaneously from the top of a 100 m high tower. Stone B is projected horizontally with speed 10 m/s, and A is dropped from the tower. Find (a) time of flight of two stones (2√2 sec) (21) A ball is thrown horizontally from the top of a tower and strikes the ground in 3 sec at an angle of 30° with the vertical. Find (a) The height of the tower (45m)
|
Conceptual Short Questions(1) Can there be motion in two dimensions with an acceleration only in one dimension? (2) Is the rocket in flight an example of a projectile? Is this a 2D motion? (3) Can the direction of the velocity of a body change when the acceleration is constant? (4) A skilled gunman always keeps his gun slightly tilted above the line of slight while shooting. Why? (5) A stone is thrown vertically upwards and then it returns to the thrower. Is it a projectile? (6) What will be the effect on the horizontal range of a projectile when its initial velocity is doubled, keeping the angle of projection the same? (7) The magnitude and direction of the acceleration of a body are constant. Will the path of the body necessarily be s straight line? (8) Why does a projectile fired along the horizontal not follow a straight line path? (9) Is it important in the long jump that how much height you take for jumping? What factors determine the span of a jump? (10) At what point in its trajectory does a projectile have its (a) minimum speed (b) maximum speed (11) A body projected horizontally moves with the same horizontal velocity although it is under the action of force( acceleration) of gravity. Why? (12) What is the angle between the direction of velocity and acceleration at the highest point of a projectile path? (13) A bullet is dropped from a certain height and at the same time, another bullet is fired horizontally from the same height, which one will hit the ground earlier and why? (14) A projectile is fired at an angle of 15° to the horizontal with the speed v, If another projectile is projected with the same speed, then at what angle with the horizontal it must be projected so as to have the same range? (15) A body is dropped freely from the window of the train. Will the time of the free fall be equal, if (a) The train is stationary (b) The train moves with a constant velocity (c) The train moves with acceleration? (16) Is the maximum height attained by a projectile is largest when its horizontal range is maximum? (17) A body is projected with speed u at an angle to have a maximum range. What is the velocity at the highest point? (18) A body slides down a smooth inclined plane when released from the top, while another body falls freely from the same point. Which one will strike the ground earlier? (19) What will be the effect on the maximum height of a projectile when its angle of projection is changed from 30° to 60°, keeping the same initial velocity of projection? ( max height becomes 3 times) (20) What is the angle of projection for a projectile motion whose range R is n times the maximum height H? (21) A projectile of mass m is fired with velocity v at an angle θ with the horizontal. What is the change in momentum as it rises to the highest point of the trajectory? (22) A person sitting in a moving train throws a ball vertically upward. How does the ball appear to move to an observer (a) inside the train and (b) outside the train? (23) A projectile of mass m is thrown with velocity v from the ground at an angle of 45° with the horizontal. What is the magnitude of change in momentum between leaving and arriving back at the ground? (24) The greatest height to which a man can throw a stone is h. What will be the greatest distance up to which he can throw the stone? (2h) (25) Why does a tennis ball bounce higher on hills than in plain? (26) A stone is thrown horizontally with a speed (27) Two projectiles A and B are projected with velocities √2 v and v respectively. They have the same range. If A is thrown at an angle of 15° with the horizontal, then what is the angle of projection of B. |

 If all have the same speed, then prove that
If all have the same speed, then prove that